Turbulent Flow Over A Backward Facing Step

In this example, I will be using the open source solver, simpleFOAM to solve the two-dimensional turbulent flow over a backward-facing step with a Reynolds number of Re = 5100. This Reynolds number is based on the step height, h = 0.1m as shown in Figure 1. I will be using the incoming velocity $u_{x} = 1m/s$ , $u_{y} = 0 m/s$ , and $\rho = 1 kg/m^3$ yielding $\mu = 1.96x10^{-5} N.s/m^2$ for the dynamic viscosity. I will be utilizing three different turbulent models Spalart-Alamars, $k-\epsilon$ , and $k-\omega$ SST with the aim to test each model’s accuracy against other numerical data. From extremely accurate direct numerical simulations (DNS), it has been determined that the reattachment of the separated boundary layer occurs on the bottom wall at $x = 16.28h = 1.628m$ .

problem1

Figure 1: Geometry for turbulent flow over a backward-facing step.

Here are the sections of this post:

Case-setup and Boundary Conditions
Grid convergence study
Results and Validation
Conclusion
Some Useful References

Download the case file here: SA-CaseFile

Assumptions

Incompressible flow
Turbulent flow
Newtonian flow
2-Dimensional flow
Negligible gravitational effects
Sea level conditions
RANS turbulence modelling without wall functions

Case Setup and Boundary Conditions

The boundary specifications are; an incoming turbulent intensity of 10% which corresponds to a turbulent kinetic energy of $k=0.015m^2/s^2$ at the inlet. The outlet boundary is defined as an outflow condition while the no-slip condition is invoked for the rest of the computational walls. Show convergence histories, pressure contours for the entire flow region, and plot velocity vectors or pathlines. Discuss any observed flow characteristics. Use grids of varying densities to determine the sensitivity of the mesh to the reattachment point of the recirculation zone. Perform a grid convergence study using the reattachment location as the target quantity. Employ at least two different turbulence models.

BCBFS

Figure 2: quadrilateral mesh.

k-epsilon Boundary Conditions Setup

BWFSepsi

Calculations of $k$ and $\epsilon$

The turbulent kinetic energy, $k$ , and turbulent dissipation rate, $\epsilon$ , were calculated as:

$k=\dfrac{3}{2}\left( u_{avg}\times I\right)^{2}$

where $u_{avg}$ is the mean velocity inlet. For turbulent intensity of $10\%$ , the turbulent kinetic energy is $k=0.015m^2/s^2$ . The turbulent dissipation rate, $\epsilon$ , is calculated as:

$\epsilon=\dfrac{0.09k^{2}}{\beta \nu}$

where $\beta$ is the turbulent viscosity ratio. For internal flows $\beta$ may be scaled with the Reynolds numbers. In our case, for $Re=5100$ , $\beta \simeq 16.5$ . Therefore, $\epsilon=0.06262 m^2 / s^3$ .

SA Boundary Conditions Setup

BWFSSA

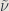
Calculations of $\nu_{t}$ and $\tilde{\nu}$

The turbulent eddy viscosity is computed from:

$\nu_{t}=f_{\nu1} \tilde{\nu}$

where

$f_{\nu1}=\dfrac{\chi^{3}}{\chi^{3}+C_{\nu1}^{3}}$

where $f_{\nu1}$ is a viscous damping function and $latex C_{\nu 1}$ is a constant equal to 7.1.

$\chi=\frac{\tilde{\nu}}{\nu}$

where $\chi=\beta$ and $\beta$ is the turbulent viscosity ratio, which is simply the ratio of turbulent to laminar (molecular) viscosity. For internal flows $\beta$ may be scaled with the Reynolds numbers. In our case, for $Re=5100$ , $\beta \simeq 16.5$ . Therefore,

$\chi=\beta=16.4=\frac{\tilde{\nu}}{\nu} \Rightarrow \tilde{\nu}=3\nu=3.234\times 10^{-5} m^{2}/s$

$\nu_{t}=\tilde{\nu}f_{\nu1}=\tilde{\nu}\dfrac{\chi^{3}}{\chi^{3}+C_{\nu1}^{3}}=2.99\times 10^{-5} m^{2}/s$

k-omegaSST Boundary Conditions Setup

BWFSOmega

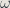
Calculations of $k$ and $\omega$

The turbulent kinetic energy, $k$ , and specific dissipation rate, $\omega$ , were calculated as:

$k=\dfrac{3}{2}\left( u_{avg}\times I\right)^{2}$

where $u_{avg}$ is the mean velocity inlet. For turbulent intensity of $10\%$ , the turbulent kinetic energy is $k=0.015m^2/s^2$ . The turbulent dissipation rate, $\epsilon$ , is calculated as:

$\epsilon=\dfrac{0.09k^{2}}{\beta \nu}$

where $\beta$ is the turbulent viscosity ratio. For internal flows $\beta$ may be scaled with the Reynolds numbers. In our case, for $Re=5100$ , $\beta \simeq 16.5$ . Therefore, $\epsilon=0.06262 m^2 / s^3$ .

Grid Convergence Study

Three grids were made with number of nodes 7063, 29000, and 116248 respectively. According to NASA calculator for Re =5100 and ref. length 0.1, mesh spacing for the fine mesh should be 0.0002529. Mesh spacing for both the coarse mesh and medium were 0.0007529 and 0.0005529 respectively. Bases on the three grids, a grid convergence study was performed for each turbulent model to see how the results would vary. The three meshes used for $k-\omega$ SST model are shown in Figure (3). Table 4 provides the grid convergence study results for $k-\omega$ SST model.

GCSBFS

GCSBFSFit

Coars Mesh with 0.0007529-7063 — Coarse grid

Coars Mesh with 0.0007529-7063zoom — Closer view of the coarse grid.

Medium mesh with 0.0005529 — Medium grid

Medium mesh with 0.0005529zoom — Closer view of the medium grid.

fine mesh with 0.0002529-116248 — Fine grid

fine mesh with 0.0002529-116248zoom — Closer view of the fine grid.

Figure 3: Shows coarse, medium, and fine mesh used for $k-\omega$ SST model.

Results and Verification

k-epsilon v dis.

k-omega v dis.

SA v dis.

SA p dis.

k-omega p dis.

k-epsilon p dis.

Figure 4: Shows the pressure and velocity distributions for each turbulent models used for the simulation.

From extremely accurate direct numerical simulations (DNS), it has been determined that the reattachment of the separated boundary layer occurs on the bottom wall at $x = 16.28h = 1.628m$ (note the origin of the coordinate system)

VeriBWFS

Conclusion

simpleFOAM has been verified for a classic example of an internal flow of a backward-facing step using three turbulent models in order to compare the results and how they affect the quantity of interests i.e. reattachment point. It has shown a great convergence study for the reattachment point. Boundary conditions for Spalat-Allmaras have been set up properly to resolve the boundary layer as well as $k-\epsilon$ with wall function (i.e. $y^{+}>30$ ) and $k-\omega$ SST without wall function. Furthermore, a comparison between DNS and simpleFOAM for the reattachment point show reasonable agreements.

Assumptions

Case Setup and Boundary Conditions

k-epsilon Boundary Conditions Setup

SA Boundary Conditions Setup

k-omegaSST Boundary Conditions Setup

Grid Convergence Study

Results and Verification

Conclusion

Some Useful References

Share this:

Like this:

Related

Leave a Reply Cancel reply